Teaching Physics with the Physics Suite Edward F. Redish Home | Action Research Kit| Sample Problems | Resources | Product Information
	Problems Sorted by Type | Problems Sorted by Subject | Problems Sorted by Chapter in UP

A long taut spring is started at a time t=0 with a pulse moving in the +x direction in the shape given by the function f(x) with (The units of x and f are in centimeters.) 

1. Draw a labeled graph showing the shape of the string at t = 0.

2. If the mass density of the string is 50 grams/meter and it is under a tension of 5 Newtons, draw a labeled graph that shows the shape and position of the string at a time t = 0.001 s.

3. Write the solution of the wave equation that explicitly gives the displacement of any piece of the string at any time.

4. What is the speed of a piece of string that is moving up after the pulse has reached it but before it has risen to its maximum displacement? What is its speed while it is returning to its original position after the pulse's peak has passed it?

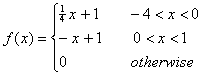
Not finding what you wanted? Check the Site Map for more information.

Page last modified October 30, 2002: O22