Problems for Intermediate Methods in Theoretical Physics Edward F. Redish

The diffusion equation for the motion of a small particle in a fluid is

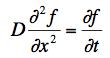
where D is a diffusion constant and f(x,t) is the number density of small particles per unit volume at the position x at a time t.

(a) From our experience with differential equations, we expect that f might be an exponential. But an exponential has to be a function of a dimensionless variable. Create a dimensionless variable, z, by combining the dimensioned space and time variables with the diffusion constant.

(b) Let us model our small particles as tiny spheres of radius a. We expect that their jiggling around and subsequent diffusion is a result of the molecules of the fluid bumping into them in random ways. We also expect that their ability to get through the fluid depends on the resistance of the fluid. Let us model the fluid resistance by a viscous force, F_visc = -bv, and note that the temperature is related to an energy by Boltzmann's constant,Θ = k_BT. Express the diffusion constant D in terms of the lower-level system parameters a, b, and Θ.

(c) The diffusion constant, D , is a measure of how fast the small particles spread out; bigger D means more diffusion, smaller D less. From your results above, does the system behave as you expect as the temperature, T , increases? As the viscosity coefficient, b , increases? Explain.

Solution

(a) In the diffusion equation, in order for the dimensions to work out, the dimensions of D have to be [L²/T]. (Note that the dimensions of f do not matter. Since it occurs on both sides of the equation, its dimensions will camcel. We are instructed to create a dimensionless variable z out of the constant D and the variables x and t. This is straightforward.

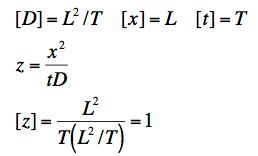
(b) Again, we do this by dimensional analysis. The dimensions of the relevant constants are:

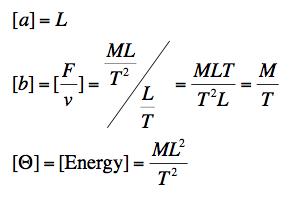
To make a combination with the dimensions of D we can write a set of dimensional analysis equations:

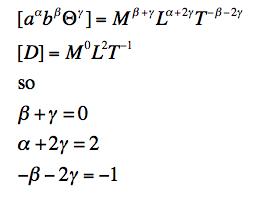
The results are easily found: α = 0, β = -1, γ = 1, so

.

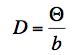(c) As T increases, so does Theta so D gets larger. This makes sense. At a higher temperature there will be more vigorous jiggling so the small particles should drift more quickly. As b increases, the drag increases so we would expect the small particles to not be able to move as quickly and, indeed, as b increases D will decrease since b is in the denominator.

RETURNS

University of Maryland	Physics Department	Physics 374 Home

Last revision 8. November, 2005.