


Next: About this document ...
Up: Homework Solutions for PHYS262,
Previous: Homework Solutions for PHYS262,
- 1-1
- Generally a simple harmonic oscillation can be expressed as
 , and the coefficients
, and the coefficients
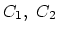 are determined by the initial condition (at
are determined by the initial condition (at 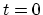 , for
instance). (a) By taking
, for
instance). (a) By taking 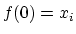 and
and
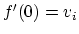 , you can
show that
, you can
show that  and
and
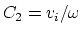 . (b) Just an algebraic
(trigonometric) identity.
. (b) Just an algebraic
(trigonometric) identity.
- 1-2
- The wave motion is
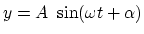 , the speed is
, the speed is
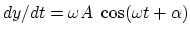 , the acceleration
, the acceleration
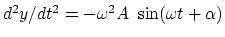 . Here
. Here
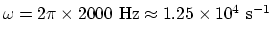 ,
,
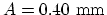 .
(a) The maximum
acceleration =
.
(a) The maximum
acceleration =
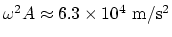 ,
and the max speed
,
and the max speed
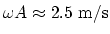 . (b) When
. (b) When
 , the acceleration=
, the acceleration=
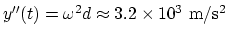 ,
and the speed=
,
and the speed=
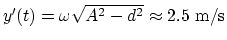 from the
identity
from the
identity
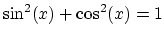 .
.
- 1-3
- This is a real-life example of a mass-spring system. (a)
The total mass at the end of the spring is
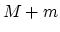 , and so the
relationship between the period
, and so the
relationship between the period 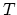 , spring constant
, spring constant 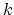 , and the total mass is
, and the total mass is
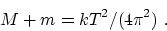
(b) In this case,
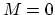
, so
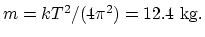
(c)
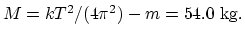
- 1-4
-
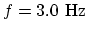 . (a)
. (a)
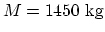 . Then
. Then
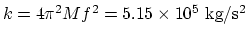 . (b) An additional mass
. (b) An additional mass
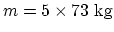 is added to the system. The result is
is added to the system. The result is
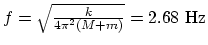 .
.
- 1-5
- (a) For S.H.O.,
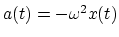 . So
. So
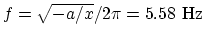 . (b)
. (b)
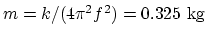 .
(c) From the relation
.
(c) From the relation
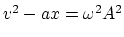 ,
,
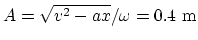 .
.
- 1-6
- Given info:
 ,
,
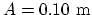 , and
, and
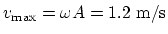 .
Firstly, the frequency is just
.
Firstly, the frequency is just
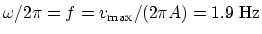 . Secondly, from the known energy and the frequency,
. Secondly, from the known energy and the frequency,
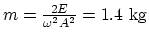 . Lastly,
. Lastly,
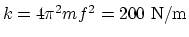 .
.
- 1-7
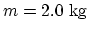 and
and
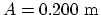 .
(a)
.
(a)
 . (b)
. (b)
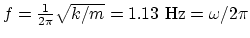 . (c)
. (c)
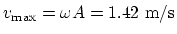 . (d)
. (d)
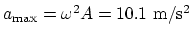 . (e)
. (e)
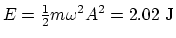 . Now if
. Now if 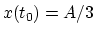 at
certain
at
certain 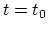 , (g)
, (g)
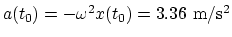 . (f)
. (f)
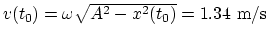 .
.
- 1-8
- This is a physical pendulum with a moment of inertia
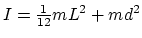 (note: parallel axis theorem)
with a gravitational force (torque) action at a distance
(note: parallel axis theorem)
with a gravitational force (torque) action at a distance 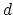 .
Then the small amplitude period of oscillation is
.
Then the small amplitude period of oscillation is
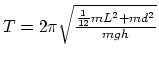 .
.
- 1-9
- You first have to understand that the constant
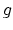 in the
formula
in the
formula
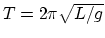 is actually the total effective
acceleration on the mass
is actually the total effective
acceleration on the mass 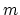 . Inside a frame with an upward
acceleration
. Inside a frame with an upward
acceleration 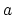 , the total effective
acceleration is
, the total effective
acceleration is
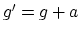 . (a)
. (a)
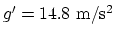 and
and
 . (b)
. (b)
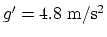 , and
, and
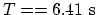 (c) The effective
acceleration is obtained by vector addition
(c) The effective
acceleration is obtained by vector addition
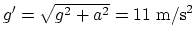 , and
, and
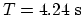 .
.
- 1-10
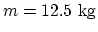 and
and
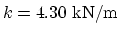 .
(a) The natural frequency of the system is
.
(a) The natural frequency of the system is
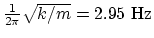 . (b) The physical contact between the mass and the
spring is minimal (zero) when the downward acceleration is equal to
the gravitation.
. (b) The physical contact between the mass and the
spring is minimal (zero) when the downward acceleration is equal to
the gravitation.
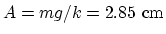 .
.


 Next: About this document ...
Up: Homework Solutions for PHYS262,
Previous: Homework Solutions for PHYS262,
Hyok-Jon Kwon
Next: About this document ...
Up: Homework Solutions for PHYS262,
Previous: Homework Solutions for PHYS262,
Hyok-Jon Kwon
2001-07-22
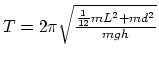 .
.
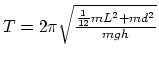 .
.